What Is The Change In The Potential Energy Of A Single Electron

18.2: Electrical potential

Last updated
Save as PDF

Page ID: 19496

Director of Outreach Technology and Senior Data Processing at University of Wisconsin-Madison

As you recall, we defined the electric field, \(\vec E(\vec r)\), to be the electric strength per unit of measurement charge. By defining an electrical field everywhere in space, nosotros were able to hands decide the force on any exam charge, \(q\), whether the test charge is positive or negative (since the sign of \(q\) will change the direction of the strength vector, \(q\vec Due east\)): \[\begin{aligned} \vec East(\vec r) &= \frac{\vec F^E(\vec r)}{q}\\ \therefore \vec F^E(\vec r)&=q\vec Eastward(\vec r)\end{aligned}\] Similarly, nosotros define the electric potential, \(V(\vec r)\), to be the electric potential energy per unit charge. This allows united states of america to define electrical potential, \(V(\vec r)\), everywhere in space, so determine the potential energy of a specific charge, \(q\), by simply multiplying \(q\) with the electric potential at that position in space. \[\brainstorm{aligned} V(\vec r) &= \frac{ U(\vec r)}{q}\\ \therefore U(\vec r)&= q 5(\vec r)\end{aligned}\] The S.I. unit for electric potential is the "volt", (V). Electric potential, \(Five(\vec r)\), is a scalar field whose value is "the electric potential" at that position in infinite. A positive charge, \(q=1\text{C}\), will thus accept a potential energy of \(U=x\text{J}\) if it is located at a position in space where the electric potential is \(V=10\text{V}\), since \(U=qV\). Similarly, a negative charge, \(q=-1\text{C}\), will take negative potential energy, \(U=-10\text{J}\), at the same location.

Since only differences in potential energy are physically meaningful (as change in potential energy is related to work), just changes in electrical potential are physically meaningful (as electric potential is related to electric potential free energy). A difference in electric potential is commonly called a "voltage". I frequently makes a clear pick of where the electrical potential is goose egg (typically the ground, or infinitely far abroad), then that the term voltage is used to describe potential, \(V\), instead of difference in potential, \(\Delta V\); this should only be done when it is clear where the location of zero electric potential is defined.

We can describe a free-falling mass by stating that the mass moves from a region where it has loftier gravitational potential free energy to a region of lower gravitational potential energy under the influence of the force of gravity (the strength associated with a potential energy always acts in the direction to decreases potential free energy). The same is true for electrical potential energy: charges will ever experience a force in a direction to decrease their electrical potential free energy. Even so, positive charges will experience a force driving them from regions of high electrical potential to regions of low electric potential, whereas negative charges will experience a strength driving them from regions of low electrical potential to regions of higher electrical potential. This is because, for negative charges, the change in potential energy associated with moving through space, \(\Delta U\), will be the negative of the corresponding change in electric potential, \(\Delta U=q\Delta V\), since the charge, \(q\), is negative.

Exercise \(\PageIndex{i}\)

Electric potential increases along the \(x\) centrality. A proton and an electron are placed at rest at the origin; in which direction do the charges move when released?

the proton moves towards negative \(x\), while the electron moves towards positive \(x\).
the proton moves towards positive \(ten\), while the electron moves towards negative \(10\).
the proton and electron move towards negative \(x\).
the proton and electron motion towards positive \(x\).

Answer

If the merely force exerted on a particle is the electrical force, and the particle moves in space such that the electric potential changes by \(\Delta V\), we tin use conservation of energy to determine the respective change in kinetic energy of the particle:

\[\begin{aligned} \Delta E&=\Delta U+\Delta Grand=0 \\ \Delta U&=q\Delta Five \end{aligned}\]

\[\therefore \Delta Yard=-q\Delta 5\]

where \(\Delta E\) is the modify in full mechanical energy of the particle, which is zero when free energy is conserved. The kinetic energy of a positive particle increases if the particle moves from a region of high potential to a region of low potential (as \(\Delta 5\) would exist negative and \(q\) is positive), and vice versa for a negative particle. This makes sense, since a positive and negative particle feel forces in opposite directions.

In guild to describe the energies of particles such equally electrons, it is convenient to employ a different unit of energy than the Joule, so that the quantities involved are not orders of magnitude smaller than i. A mutual choice is the "electron volt", . One electron volt corresponds to the energy caused past a particle with a charge of \(e\) (the accuse of the electron) when it is accelerated by a potential difference of \(i\text{5}\): \[\begin{aligned} \Delta E &= q\Delta 5\\ 1\text{eV}&=(due east)(1\text{V})=1.half-dozen\times 10^{-19}\text{J}\finish{aligned}\] An electron that has accelerated from residue across a region with a \(150\text{V}\) potential divergence across information technology will accept a kinetic of \(150\text{eV}=2.4\times 10^{-17}\text{J}\). As you tin run into, it is easier to draw the free energy of an electron in electron volts than Joules.

Exercise \(\PageIndex{ii}\)

A particle moves from an electric potential of \(-260\text{ 5}\) to an electric potential of \(-600\text{ V}\) and loses kinetic energy. What is the accuse of this particle?

Neutral.
Information technology could accept a positive or a negative accuse.
Positive.
Negative.

Reply

josh'due south thoughts

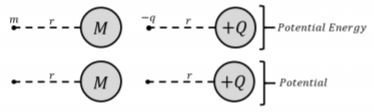
It is often useful in physics to take previously learned concepts and compare them to new ones, in this instance, gravitational potential energy and electric potential energy tin be compared to help understand the physical meaning of electric potential.

Suppose that an object with a large mass, \(M\), is sitting in space. Now identify an object of a much smaller mass, \(one thousand\), at any distance, \(r\), from the eye of \(M\). The gravitational potential energy of the pocket-size mass is given past the post-obit formula: \[\brainstorm{aligned} U_g&=\frac{GMm}{r}\finish{aligned}\] Which is very similar to the formula for electrical potential energy: \[\begin{aligned} U(\vec r)&=\frac{kQq}{r}\end{aligned}\] Now, if nosotros were to remove the mass \(m\) from its position, we would no longer accept an object with gravitational potential energy. Withal, nosotros could notwithstanding draw the gravitational potential for the bespeak, \(r\), which would result in gravitational potential energy when any mass \(m\) is placed there. This is the gravitational equivalent to electric potential, and can be defined as: \[\begin{aligned} V_g&=\frac{U_g}{thousand}\end{aligned}\] which is as well very similar to the formula for electric potential: \[\brainstorm{aligned} V_E&=\frac{U_E}{q}\end{aligned}\] This comparison is illustrated in Figure \(\PageIndex{1}\).

Figure \(\PageIndex{1}\): Gravitational potential free energy and gravitational potential (left) adjacent to its electrical analogue (right).

Example \(\PageIndex{one}\)

A proton and an electron move from a region of infinite where the electric potential is \(twenty\text{V}\) to a region of infinite where the electric potential is \(10\text{5}\). If the electric forcefulness is the only forcefulness exerted on the particles, what can you lot say nigh their modify in speed?

Solution:

The two particles move from a region of space where the electric potential is \(20\text{V}\) to a region of space where the electric potential is \(x\text{Five}\). The change in electric potential experienced past the particles is thus: \[\begin{aligned} \Delta V = V_{concluding}-V_{initial}=(10\text{V})-(twenty\text{V})=-10\text{Five}\end{aligned}\] and we have the opportunity to emphasize that one should be very conscientious with signs when using potential. The modify in potential energy of the proton, with charge \(q=+e\), is thus: \[\begin{aligned} \Delta U_p=q\Delta 5 = (+eastward)(-10\text{V})=-10\text{eV}\stop{aligned}\] The potential free energy of the proton thus decreases by \(10\text{eV}\) (which y'all can hands convert to Joules). Since we are told that no other strength is exerted on the particle, the full mechanical free energy of the particle (kinetic plus potential energies) must be constant. Thus, if the potential free energy decreased, then the kinetic free energy of the proton has increased past the same amount, and the proton'southward speed increases.

The alter in potential energy of the electron, with accuse \(q=-e\), is thus: \[\brainstorm{aligned} \Delta U_e=q\Delta V = (-e)(-10\text{5}) = 10\text{eV}\end{aligned}\] The potential free energy of the electron thus increases by \(x\text{eV}\). Once again, the mechanical energy of the electron is conserved, and then that an increase in potential energy results in the same decrease in kinetic energy and the electron'southward speed decreases.

Word:

By using the electric potential, \(Five\), nosotros modelled the modify in electric potential energy of a proton and an electron as they both moved from i region of space to another.

We institute that when a proton moves from a region of high electric potential to a region of lower electric potential, its potential energy decreases. This is considering the proton has a positive charge and a decrease in electric potential will also result in a decrease in potential energy. Since no other forces are exerted on the proton, the proton's kinetic energy must increase. Because the potential energy of the proton decreases, the proton is moving in the same direction equally the electrical force, and the electric strength does positive work on the proton to increment its kinetic energy.

Conversely, we found that when an electron moves from a region of high electrical potential to a region of lower electric potential, its potential energy increases. This is because information technology has a negative charge and a decrease in electrical potential thus results in an increase in potential energy. Since no other forces are exerted on the electron, the electron'south kinetic energy must decrease, and the electron slows down. This makes sense, since the force that is exerted on an electron volition be in the contrary direction from the force exerted on a proton.

Electrical potential from electric field

At the beginning of Section 18.one, nosotros determined the potential energy of a point accuse, \(q\), in the presence of some other point charge, \(Q\) (Figure \(\PageIndex{1}\)). This was done by calculating the work washed past the Coulomb (electric) forcefulness exerted by accuse \(Q\) on \(q\). We can write the aforementioned integral for the work washed by the electric force on \(q\), merely using the electric field, \(\vec E\), to write the strength: \[\begin{aligned} Westward&=\int_A^B \vec F^E\cdot d\vec r=\int_A^B q \vec Due east\cdot d\vec r=q \int_A^B \vec E\cdot d\vec r\cease{aligned}\] where nosotros recognized that the charge, \(q\), is constant and can come out of the integral. The integral that is left is thus the piece of work done by the electric field, \(\vec Due east\), per unit charge. In other words, this is the negative modify in electric potential:

\[\begin{aligned} W=q\int_{A}^{B}\vec Eastward\cdot d\vec r=-q\Delta V=-q[Five(\vec r_{B})-V(\vec r_{A})] \cease{aligned}\]

\[\therefore\Delta V=V(\vec r_{B})-V(\vec r_{A})=-\int_A^B\vec E\cdot d\vec r\]

which allows us to easily make up one's mind the change in electric potential associated with an electric field. Notation that this result is general and does non crave the electric field to be that of a signal charge, and can exist used to determine the electric potential associated with any electric field. We can also specify a function for the potential, up to an arbitrary constant, \(C\), (call back definite versus indefinite integrals): \[\begin{aligned} V(\vec r)=-\int \vec E\cdot d\vec r + C\terminate{aligned}\] The relation between electric potential and electric field is analogous to the relation between electric potential free energy and electric force: \[\brainstorm{aligned} \Delta V &=V(\vec r_B)-Five(\vec r_A)=-\int_A^B \vec E\cdot d\vec r\\ \Delta U &=U(\vec r_B)-U(\vec r_A)=-\int_A^B \vec F^E\cdot d\vec r\finish{aligned}\] as the bottom equation is just \(q\) times the first equation. We tin think of electric potential being to potential energy what electric field is to electrical force. Electrical potential and electrical field are electric potential energy and electric forcefulness, per unit charge, respectively.

For a signal charge, \(Q\), located at the origin, the electric field at some position, \(\vec r\), is given by Coulomb'southward Law: \[\begin{aligned} \vec E=\frac{kQ}{r^2}\hat r\finish{aligned}\] The potential difference between location \(A\) (at position \(\vec r_A\)) and location \(B\) (at position \(\vec r_B\)), as in Figure \(\PageIndex{1}\), is given past: \[\begin{aligned} \Delta 5 &=- \int_A^B \vec E\cdot d\vec r= -\int_{\vec r_A}^{\vec r_B} \frac{kQ}{r^2}\hat r\cdot d\vec r=-\left(\frac{kQ}{r_B}-\frac{kQ}{r_A}\right)\end{aligned}\] and we note that we can write a role for the electric potential, \(V(\vec r)\), at a distance \(r\) from a point accuse, \(Q\), as: \[\begin{aligned} 5(\vec r)=\frac{kQ}{r}+C\end{aligned}\] where \(C\) is an capricious constant. This, of grade, is identical to the result that we obtained before, for the potential free energy of a charge, \(q\), a distance, \(r\), from \(Q\). \[\begin{aligned} U(\vec r)=qV(\vec r)=\frac{kQq}{r}+C'\end{aligned}\] where the constant, \(C'=qC\), does not accept any physical bear on. Oftentimes, as is the case for gravity, one chooses the constant \(C=0\). This selection corresponds to defining potential energy to be zero at infinity. Equivalently, this corresponds to choosing infinity to be at an electrical potential of \(0\text{V}\).

Exercise \(\PageIndex{3}\)

What causes a positively charged particle to gain speed when it is accelerated through a potential difference?:

The particle accelerates because it loses potential energy as it moves from loftier to low potential.
The particle accelerates considering information technology loses potential free energy as it moves from depression to high potential
The particle accelerates because it gains potential energy.
The particle accelerates considering information technology moves towards negative charges.

Answer

Case \(\PageIndex{2}\)

What is the electric potential at the border of a hydrogen atom (a distance of \(one\) from the proton), if one sets \(0\text{ Five}\) at infinity? If an electron is located at a distance of \(1\) from the proton, how much energy is required to remove the electron; that is, how much energy is required to ionize the hydrogen atom?

Solution:

We can easily calculate the electric potential, a altitude of \(1\unicode{xC5}\) from a proton, since this corresponds to the potential from a bespeak charge (with \(C=0\)): \[\begin{aligned} V(\vec r)=\frac{kQ}{r}=\frac{(nine\times 10^{9}\text{N}\cdot\text{m}^ii\text{/C}^{2})(i.6\times 10^{-19}\text{C})}{(i\times x^{-10}\text{m})}=14.4\text{Five}\end{aligned}\] We can calculate the potential energy of the electron (relative to infinity, where the potential is \(0\text{ Five}\), since we chose \(C=0\)): \[\begin{aligned} U=(-e)5=(-ane.six\times 10^{-19}\text{C})(xiv.4\text{V})=-14.4\text{eV}=-2.3\times 10^{-18}\text{J}\end{aligned}\] where nosotros besides expressed the potential energy in electron volts. In lodge to remove the electron from the hydrogen cantlet, nosotros must exert a force (exercise piece of work) until the electron is infinitely far from the proton. At infinity, the potential free energy of the electron volition be zero (by our choice of \(C=0\)). When moving the electron from the hydrogen atom to an infinite distance abroad, we must do positive work to counter the attractive forcefulness from the proton. The work that we must do is exactly equal to the change in potential energy of the electron (and equal to the negative of the work done past the force exerted by the proton):

\[\begin{aligned} W=\Delta U=(U_{final}-U_{initial})=(0\text{J}--2.iii\times 10^{-eighteen}\text{J})=2.iii\times 10^{-18}\text{J} \stop{aligned}\]

The positive work that nosotros must practice, exerting a strength that is opposite to the electric force, is positive and equal to \(2.iii\times x^{-18}\text{J}\), or \(14.iv\text{eV}\). If you look up the ionization energy of hydrogen, y'all will find that it is \(13.six\text{eV}\), so that this very simplistic model is quite accurate (we could better the model past adjusting the proton-electron distance so that the potential is \(xiii.six\text{5}\)).

Discussion:

In this example, we determined the electrical potential energy of an electron in a hydrogen atom, and found that it is negative, when potential energy is defined to be zero at infinity. In order to remove the electron from the atom, we must do positive work in order to increase the potential energy of the electron from a negative value to zero (the potential free energy at infinity). This is analogous to the work that must be done on a satellite in a gravitationally bound orbit for it to reach escape velocity.

Example \(\PageIndex{three}\)

Two large parallel plates are separated by a distance, \(50\). The plates are oppositely charged and carry the same magnitude of rate area, \(\sigma\). What is the potential divergence between the two plates? Write an expression for the electric potential in the region betwixt the two plates. Assume that the plates are large enough that you lot can care for them as infinite (that is, neglect what happens almost the edges).

Solution:

Figure \(\PageIndex{ii}\) shows a diagram of the two parallel plates with surface charge on them.

Effigy \(\PageIndex{ii}\): Two parallel plates with equal and opposite surface charge densities. In the region betwixt the plates, the electric field is compatible.

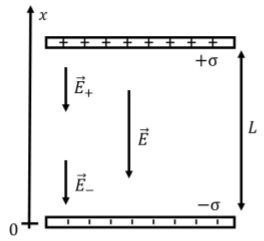
We know from the previous capacity that the electric field from the positive plate does non depend on distance from the plate and is given by: \[\begin{aligned} \vec E_+=-\frac{\sigma}{two\epsilon_0} \lid x\end{aligned}\] if we guess the plate every bit existence infinitely large. This is a reasonable approximation for most points except those near the edges of the plate, which we ignore. The electric field from the negative plate will take the same magnitude and direction, so that the total electric field, \(\vec E\), everywhere between the 2 parallel plates (every bit long as we are not near the edges) is given by: \[\begin{aligned} \vec E=-\frac{\sigma}{\epsilon_0} \hat x\end{aligned}\] Note that the electric field exterior the region between the 2 plates is nothing everywhere, as the field from the positive and negative plates point in opposite directions exterior the plates and thus cancel (except near the edges of the plates). For instance, beneath the negative plate, the field from the negative plate points in the positive \(x\) management (towards the negative plate), whereas the field from the positive plate points in the positive \(x\) direction (towards the positive plate).

We can now make up one's mind the potential difference between the two plates, since we know the electrical field in that region. Using the coordinate system that is shown, we calculate the potential difference between the positive plate located at \(ten=L\) and the negative plate located at \(x=0\): \[\begin{aligned} \Delta V &=Five(L)-V(0)=- \int_0^L \vec E\cdot d\vec x=-\int_0^50\frac{-\sigma}{\epsilon_0} \hat ten \cdot d\vec x=\frac{\sigma}{\epsilon_0}\int_0^L dx=\frac{\sigma}{\epsilon_0}L\end{aligned}\] where we recognized that \(\hat ten\) and \(d\vec ten\) are parallel. It is very easy to get the wrong sign when calculating potential differences, so exist careful!

Since the potential difference, \(\Delta 5=V(Fifty)-V(0)\), is positive, the plate at \(x=Fifty\) is at a higher electrical potential than the plate at \(ten=0\). This makes sense, as a positive charge at rest would motion from the positive plate to the negative plate, thus decreasing its potential free energy, which corresponds to moving from a region of high electric potential to a region of low electrical potential. Conversely, a negative charge at remainder would motility from the negative plate to the positive plate, decreasing its potential energy, but moving from a region of depression electric potential to a region of high electrical potential.

In general, if the electric field is constant, the change in potential betwixt 2 points separated by a distance, \(50\), along an axis that is anti-parallel with the field (in this case, the field points in the negative \(x\) management) is given by: \[\begin{aligned} \Delta V =- \int_0^L \vec East\cdot d\vec 10=E\int_0^50 dx= EL\stop{aligned}\]

Note that we tin can only calculate the deviation in electrical potential betwixt plates, not the actual value of the potential, \(5\). If we want to define a specific value of electric potential, we demand to cull a location where we define \(0\text{V}\) to be. By convention, when possible, 1 chooses the negative plate to exist the location of \(0\text{V}\). In guild to determine the electric potential anywhere between the two plates, nosotros can calculate the potential difference between the plate at \(x=0\) (the one at \(0\text{Five}\)) and some position between the plates along the \(10\) axis (\(x<L\)): \[\begin{aligned} \Delta 5 &=V(x)-V(0)=-\int_0^ten E \hat 10 \cdot d\vec 10= Ex =\frac{\sigma}{\epsilon_0}10\\ \therefore V(x)&=V(0)+Ex=Ex=\frac{\sigma}{\epsilon_0}x\end{aligned}\] where we notice that the electric potential increases linearly between its value at the negative plate (\(0\text{Five}\)) and its value at the positive plate (\(EL\)). Of form, nosotros could have chosen any value of the electrical potential for the negative plate, which is equivalent to choosing the value of the arbitrary constant, \(C\).

In general, we can write the electric potential in a region of constant electric field, \(\vec Due east=-Due east\lid ten\), every bit: \[\begin{aligned} 5(x)=Ex + C\end{aligned}\] This scenario is very like to the gravitational forcefulness near the surface of the Globe, where the gravitational field is (almost) constant. If y'all choose to define zero gravitational potential energy at the surface of the Earth, so, equally y'all move upwards a distance \(h\) from the basis, your gravitational potential free energy increases linearly with \(h\) (\(U(h)=mgh\)). In our case, we defined zip electrical potential energy to represent to the location of the negative plate (the negative plate is thus like the surface of the Earth, with a constant electric field pointing towards information technology). Every bit a positive accuse moves a altitude \(h\) away from the negative plate, it gains electric potential energy, \(U(h)=qV(h)=qEh\), linearly with distance from the plate. If we release that positive charge, information technology will "autumn" dorsum onto the negative plate. The principal departure with gravity, is that we can also have negative charges, which nether gravity, would exist similar to "negative masses" (it's non a thing), which would "fall upwards" (towards the positive plate).

Discussion:

In this case, we examined the electric field between 2 parallel plates with opposite charges on them, and saw that the field is abiding and uniform between the plates and aught outside (except for a modest region near the edge of the plates where the assumption of infinitely big plates breaks down). We found that the electric potential decreases linearly equally a office of distance from one of the plates. Because the electric field is constant between the two plates, the electric force on a charge tin can be treated in a like way as the gravitational forcefulness on a mass nearly the surface of the Globe. The resulting electric potential is linear in the distance from the negative plate, only as \(mgh\) is linear in \(h\), the distance to the surface of the World. Parallel plates are often used to accelerate charges, and then they are useful to understand.

Practice \(\PageIndex{4}\)

If we defined a gravitional potential, \(V(h)\), for particles a minor distance, \(h\), from the surface of the World, it would accept the form:

\(Five(h) = mgh + C\).
\(V(h) = gh + C\).
\(Five(h) = mg + C\).
\(V(h) = -mgh + C\).

Reply

Electric field from electric potential

review topics

Section 8.2 on determining force from potential energy.
Section A2.two on gradients.

In the previous section, nosotros found that we could decide the electric potential (a scalar) from the electric field vector. In this department, we show how to exercise the reverse, and determine the electric field vector from the electric potential. Consider, showtime, a one-dimensional case, where the electric field, \(\vec E(x)= E(x) \hat x\), point in the \(10\) direction and depends on position, \(x\). In this one-dimensional case, the electrical potential is obtained from the negative anti-derivative of the electric field: \[\begin{aligned} 5(x)=-\int \vec E(x)\cdot d\vec 10=-\int Due east(x) dx\end{aligned}\] The electric field must so exist given by the negative of the derivative of the electrical potential function: \[\begin{aligned} \vec Due east(ten) = -\frac{dV(x)}{dx}\hat x\cease{aligned}\] Notation that we tin can tell from the above that the electric field must have dimensions of electrical potential over altitude. The nigh mutual South.I. unit used to depict the electric field is \(\text{5/g}\) (Volts per meter).

This event is very similar to that obtained in Section 8.2, where we examined how one could use the scalar potential free energy, \(U(x,y,z)\), to determine the vector for the strength associated with that potential energy. The aforementioned holds for the electric force, where we can determine the electric forcefulness vector, \(\vec F\), from the electric potential energy, and similarly the electric field from the electric potential. In three dimensions, if we know the electric potential energy as a part of position, \(U(\vec r)=U(x,y,z)\), then the electric force vector is given past:

\[\brainstorm{aligned} \vec F(x,y,z) =- \nabla U=-\frac{\partial U}{\fractional x}\hat x-\frac{\partial U}{\partial y}\lid y-\frac{\partial U}{\partial z}\hat z\finish{aligned}\]

Similarly, simply using force per unit charge (i.e. electrical field) and potential energy per unit accuse (i.due east. electric potential), we discover:

\[\brainstorm{aligned} \vec East(x,y,z) = -\nabla V =-\frac{\fractional Five}{\fractional x}\chapeau x-\frac{\partial 5}{\partial y}\hat y-\frac{\partial V}{\partial z}\hat z\stop{aligned}\]

where, as you recall, \(\nabla 5\), is called the gradient of the scalar field, \(5(ten,y,z)\). The gradient is a vector that points in the management of maximal increase of the value of \(V(10,y,z)\). For a positive charge, this corresponds to the direction of maximal increase in potential energy. A positive charge volition feel a force in the opposite management (in the direction where the potential free energy decreases the fastest), and the electrical field is thus in the opposite direction from the gradient of the electric potential.

Equipotential surfaces

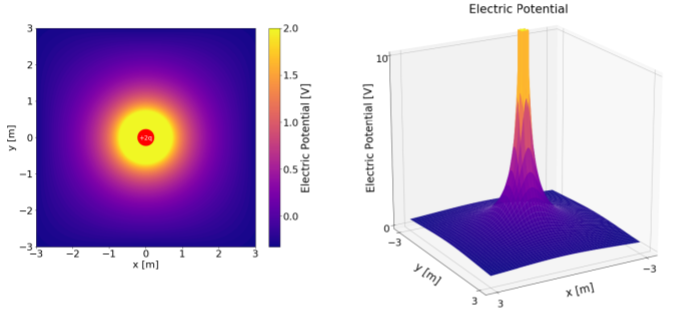
Nosotros tin can visualize electrical potential in several ways, since it is a scalar field (it has a single value that can differ everywhere in infinite). Effigy \(\PageIndex{iii}\) shows the electrical potential nigh a positive charge, \(+Q\), where one has called \(0\text{V}\) to be located at infinity. The correct panel shows the electric potential every bit a "surface plot", where the vertical direction is the value of the electric potential. The left panel shows a "oestrus map" of the electric potential, where the color corresponds to the value of the electric potential.

Figure \(\PageIndex{3}\): Electric potential heat map (left) and surface plot (right) nigh a single positive accuse.

The about common mode to visualize the electrical potential is to draw "profile lines", similar to how one draws contour lines on a geographical map. On a geographical map, contours correspond to lines of abiding altitude, which are also lines of constant gravitational potential energy. Similarly, nosotros can draw lines of constant electric potential to visualize the electrical potential. Lines of constant potential are called "equipotential lines". In general, in three dimensions, regions of constant electric potential can be surfaces or volumes, called "equipotential surfaces/volumes". In Example 18.two.3 (with the parallel plates) each of the plates forms an equipotential surface (e.g. the electric potential was fixed to \(0\text{V}\) everywhere on the negative plate).

Retrieve that, at some point in space, the electric field vector e'er points in the opposite direction of the slope of the electrical potential. Namely, the electrical field points in the direction in which the electric potential decreases the fastest. That direction must be perpendicular to the direction in which the electric potential does not change; in other words, the electric field vector is always perpendicular to equipotential lines/surfaces. More than intuitively, one tin can think about a charge moving forth an equipotential. Past definition, the electrical potential energy of the charge does not change if its moves along an equipotential. As a result, the electric strength/field cannot do whatever piece of work on the charge, and must thus be perpendicular to the path of the charge (which we chose to be an equipotential).

Conducting materials are ever equipotential surfaces (or volumes) if charges are non moving inside the conductor. The electric field inside a conductor is always zilch (in electrostatics, when charges are not moving), and thus, a accuse moving through a conductor experiences no electric forcefulness and its electrical potential energy volition be constant; in other words, the entire usher is an equipotential. Similarly, because the electrical field must always be perpendicular to an equipotential, electric field lines are always perpendicular to the surface of a conductor (in electrostatics).

In order to draw equipotential lines, one can first by drawing electric field lines, and so describe (airtight) contour lines that are everywhere perpendicular to the electric field lines. This is illustrated in Effigy \(\PageIndex{4}\).

Effigy \(\PageIndex{iv}\): The electric field and equipotential lines acquired by 2 \(+2q\) charges and one \(−3q\) charge (left) and its corresponding electric potential heatmap (right).

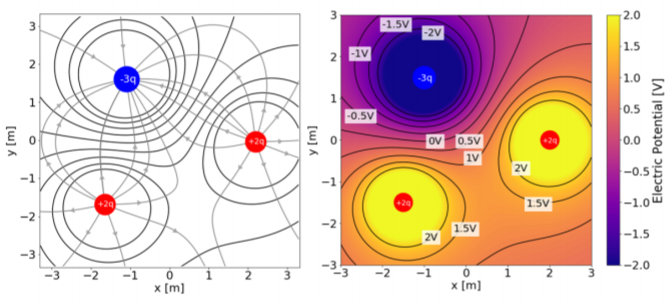
In general, it is preferable to draw equipotential lines that are separated past equal increments in electric potential (only every bit on a geographical map, the contour lines correspond to constant increments in altitude). This requires knowing a functional form for the electric potential. For case, the equipotential lines for a point charge located at the origin consist in concentric circles centerd at the origin (in 3 dimensions, this results in concentric spherical equipotential surfaces). If we define \(0\text{V}\) to be at infinity, the electric potential is given by: \[\begin{aligned} V(r)=\frac{kQ}{r}\stop{aligned}\] In lodge to depict equipotential lines every, say, \(10\text{V}\), the radii of the corresponding equipotential circles, for \(V=10\text{V}\), \(5=xx\text{Five}\), \(V=30\text{Five}\), etc., are given past: \[\brainstorm{aligned} r&=\frac{kQ}{Five}\\ r_{10V}&=\frac{kQ}{(10\text{V})}\quad r_{20V}=\frac{kQ}{(twenty\text{Five})}\quad r_{30V}=\frac{kQ}{(30\text{V})}\quad \dots\end{aligned}\]

Source: https://phys.libretexts.org/Bookshelves/University_Physics/Book%3A_Introductory_Physics_-_Building_Models_to_Describe_Our_World_(Martin_Neary_Rinaldo_and_Woodman)/18%3A_Electric_potential/18.02%3A_Electric_potential

Posted by: smithfoure1955.blogspot.com